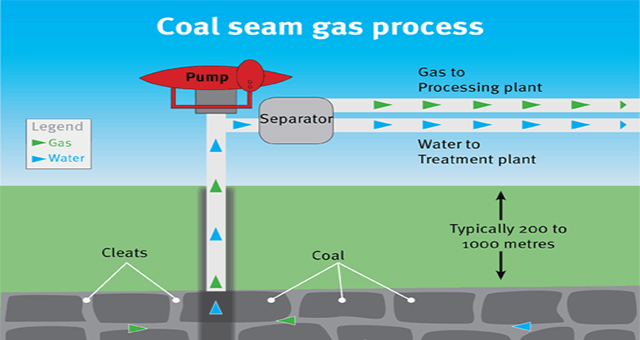
When a gas flows through a pipe, the pressure variation from one point to another is influenced by the density and the viscosity of the gas. Readily available software programs ^[1] for simulating the flow of gases through piping systems are written around single component gases of known density and viscosity. These programs may make erroneous predictions where the pumped gas is a mixture of gases for which the density and viscosity of the particular mixture have not been determined. The calculation error will increase if the component gases are significantly different in density and viscosity. An example of a gas mixture commonly pumped is coal seam gas, which is typically a mixture of air and methane in varying proportions depending on the source. (Note that although air is itself a mixture, its density and viscosity are known. Air can therefore be treated as a single component gas in the pipe-flow software.)

In Section A of this note, we present a calculation method for determining the effective density and the effective viscosity of a multi-component gas mixture from the known densities and viscosities of the component gases. Using calculated effective density and effective viscosity in pipe-flow software will enable more accurate simulations of the flow of gas mixtures.

We also present an example pressure drop calculation in Section B and compare the outcome of this with the result determined from a Computational Fluid Dynamics (CFD) simulation and an actual pressure drop measurement in the field (with the comparisons shown in Section C).

Section A: Derivation of effective density and viscosity of multi-component gas mixture

The following method may be applied to a gas mixture containing any number of component gases. For simplicity, we will present the method for a mixture of 2 gases; gas A and gas B.

We can calculate the density of each component in the mixture as follows
(1)
(2)
where and are the densities of the 2 gases; and are the respective molecular weights; is initial pressure of flow at the inlet; is universal gas constant; and is the absolute temperature.

The density of the mixture is calculated, using
(3)
where and are the mole (or volume) fractions of the components. Note that the total value of mole must be unity ( + =1).

And, the dynamic viscosity of the mixture is determined from

where and are the dynamic viscosities of the 2 gases.

Section B: Pipeline pressure drop calculation using the effective properties
The following example shows how the effective density and the effective viscosity may be used to calculate the pressure drop resulting from the flow of a gas mixture through a section of pipe. Again, for simplicity, the example derivation is provided for horizontal section of straight pipe.

Knowing the flow velocity () and diameter of the pipe (), we can determine the Reynolds number
(5)

The friction factor can be calculated using Zigrang-Sylvester equation
(6)
where is Fanning friction factor and is equivalent sand-grain roughness height of the pipe.

Finally, the pressure drop can be determined
(7)
where is the pressure drop between the measurement points in the pipe and is the distance between the two measurement points.

Section C: Comparing the theoretical method with the results from a Computational Fluid Dynamics (CFD) simulation
Another method of analysing the flow of gas through a pipe is to make a CFD simulation. In this section we will compare the pressure drop obtained by the theoretical method, with that obtained by a CFD simulation. The pressure drop is measured over a 5 metre long section of horizontal and straight 203.2 mm (8”) ID pipe transporting coal seam gas (a mixture of air and methane). The test section is assumed to be remote from entry and exit effects.
In this experiment, the distance , between pressure tappings is 5m; the mass flow at the inlet to the section is 2.6 kg/s with the mole fraction of air being 0.3 and methane 0.7; the sand-grain wall roughness, , is 0.15mm; ambient temperature () is ; ambient pressure () is 1 atm; universal gas constant, , is 8.1345 ; molecular weight of air and methane are =0.0289664 kg/mol and =0.016043 ; gauge pressure at the inlet is measured at 2.786 kPa (= 0.404 psi).

The section of pipe considered is shown below

Using the above data, the theoretical calculations as in sections A and B above compare with the CFD simulation result illustrated in Appendix 1 as follows:
Theoretical for coal gas = 1.765 kPa (= 0.256 psi)
CFD simulation for coal gas = 1.669 kPa (= 0.242 psi)
The theoretical result compares within approximately + 5% of the measured pressure drop of CFD test.
The above results compare with the following results determined using either air or methane, rather than the mixture:
Theoretical for AIR only = 1.220 kPa ( = 0.177 psi)
CFD simulation for METHANE only = 2.110 kPa ( = 0.306 psi)
As can be seen, the use of calculated gas mixture density and viscosity, rather than the individual gas properties, improves the pressure drop calculation accuracy by approximately 20% to 31%, depending on the individual gas chosen.

Concluding remarks
In this note, we have presented a calculation method that can be used to determine the effective density and effective viscosity of multi-component gas. The calculation method allows gas pipeline pressure drop software written for single component gases to provide more accurate simulations where the gas is a multi-component mixture, with a typical example being coal seam gas. It can be seen that the present analytical result is in good agreement with the CFD simulation.

Apart from pressure drop, the effective density and viscosity of the multi-gas mixture can be used for other applications where the conveyed gas is a mixture. For example, the effective density can be used in calculating mass flow rate of methane and air mixture after being pumped through a blower (given that both the pressure and temperature at the blower discharge nozzle are increased by specified values).

In this note, we include sufficient equations to enable readers to derive the effective density and viscosity of a gas mixture, given the properties of the component gases. This calculation method is provided free of any copyright restrictions. However, for users wanting to derive gas mixture properties without resorting to the mathematics, we offer a ready-to use Mathcad spreadsheet at a charge of AUD $45.00 including GST. The spreadsheet includes modifiable fields to suit your needs and can be used with the free version of Mathcad. Please contact us at info@caebay.com if you require the spreadsheet.

Appendix 1: CFD and analytical result comparison

CFD pipe geometry and meshing

CFD pressure and velocity

CFD pressure drop over a 5m length psi

Analytic pressure drop over a 5m length psi

Difference between CFD and analytical solutions Diff

 

References:
[1] Available pressure drop calculators: http://www.pressure-drop.com/Online-Calculator/, http://www.tribology-abc.com/calculators/pipeflow_fluid.htm, http://www.freecalc.com/fricfram.htm, https://www.lmnoeng.com/Pressure/PressureDrop.php, and https://www.pipeflow.com/pipe-pressure-drop-calculations
https://www.pipeflow.com/pipe-pressure-drop-calculations (accessed on 6 Jan 2019)
[2] Pipe flow calculations – Clarkson University
[3] Gas mixtures and properties – Engineering Tool Box
[4]Gas viscosity – Petro Wiki.

Acknowledgements. The author would like to thank Cliff Walker of Vacmobiles.com for his insightful comments and suggestions that greatly improved the quality of this note. Any errors that remain, of course, are the author’s own.

Copyright. CAEbay endeavours to contribute its knowledge towards engineering progress. This note is free for any use, either commercial or educational, that could make products better and safer. However, if published elsewhere, acknowledgement of the source would be much appreciated.

Disclaimer of reliability. The information in this note is correct to the best of our knowledge. However, because material supply and manufacturing quality are outside our control, all recommendations are made without guarantee on the part of the author or CAEbay. The author and CAEbay disclaim any liability in connection with the use of this information.

Feedback or queries on this note? We are keen to improve the accuracy and value of CAEbay’s notes. If you have any feedback or queries, please email info@caebay.com. We would be pleased to hear from you!